The Golden Section - Harmony of the Universe

It’s the equation hidden in nature and in objects all around us, as mysterious as the secret of its provenance - the Golden Section (or Golden Ratio) is an irrational number, a mathematical expression of perfect harmony in the universe.

Moreover, the simplicity of its construction makes it even more beautiful.

Take a line of any length (A) and divide it in two sections of different length, a and b.

A= a + b

The division of the entire length (A) by its longest section will be equal to the division of its sections:

This mathematical ‘magic’ fascinated the ancients, who studied its properties, which later became the basis for rules of geometry, algebra, architecture and more.

Although the Greek philosopher and mathematician Pythagoras was credited with the study of the Golden ratio already back in 5th Century B.C., the origin of this mathematical equation seems to be older and its roots are lost in time.

In fact, the proportions of the Great Pyramid of Giza, built around 2560 B.C., closely approximate those of a golden square pyramid.

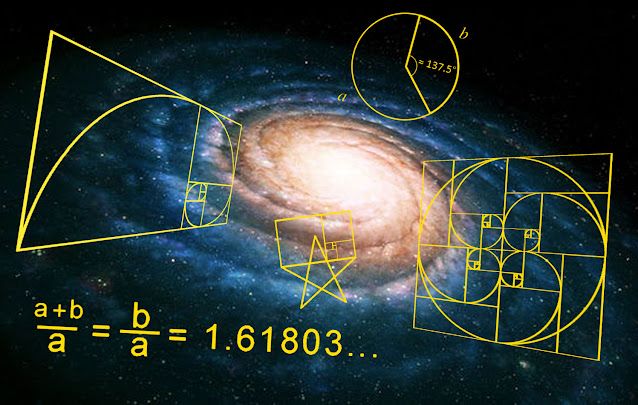
The golden ratio can be transferred to two and three dimensions, rendering thus Golden rectangles, spirals, angles, and three-dimensional solids.

When transferred to two-dimensions, the above calculation renders a square and a rectangle, which together form the ‘Golden Rectangle’.

This Golden Rectangle contains in itself endless repetitions of itself, always maintaining the same ratio of 1,61803...

When we draw curves connecting the opposite corners of each inner square, they form the ‘Golden Spiral’.

When we take the Golden ratio to a circle (the ratio of 1,61803 expressed in rotations or degrees), we get the ‘Golden Angle’.

Additionally, the ancients discovered the relationship between the proportions of a pentagram and the golden ratio.

This led ancients to believe that the Dodecahedron (the three-dimensional version of the Pentagram) was the most perfect solid in nature, the shape of the universe itself and a mystery not to be revealed to the uninitiated.

Fortunately, today we know better.

The so-called Fibonacci numbers may give us an approximation to the origins of the Golden ratio.

Leonardo Bonacci (a.k.a. Fibonacci) was an Italian mathematician of the 12th century who helped to popularize the use of Hindu-Arabic numerals in Europe (where, until then, people still worked with Roman numerals).

His exposure to Hindu-Arabic mathematics may have provided him with the clue for his mathematical progression, later called ‘Fibonacci numbers’. According to several sources, these were already present in old Indian mathematics and ancient Babylonian tablets.

The Fibonacci numbers are a simple arithmetic progression in which each number is the result of the sum of the previous two, starting with 0 and 1. Thus:

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89...

As simple as this progression may seem, its uses in mathematics, statistics, calculation of probability, computer algorithms and finance are endless and, even more surprisingly, the ratio of these numbers closely resemble that of the Golden ratio.

The higher the Fibonacci number, the closer its resemblance to the Golden ratio.

In the 16th century, the German mathematician Simon Jacob already found a relationship between the Fibonacci numbers and the Golden ratio, and this was later confirmed by Johannes Kepler in 1608.

In fact, if we see the graphic representation of the Fibonacci numbers, we can see how it closely resembles the Golden Rectangle.

Amazingly, this progression (and that of the Golden ratio) emerges spontaneously in many structures in nature, particularly those that require to compress great amounts of elements in limited space, or which require organized growth without disturbing the rest of its existing parts.

For thousands of years, people of all cultures all over the world have used this mathematical knowledge in design, architecture and the arts, since these proportions are believed to create the most pleasing and most harmonious results to the finished piece.

When we divide the Golden rectangle with two horizontal and vertical lines following its inner proportions, we get four ‘Golden points’ inside the rectangle. These points represent the section naturally favoured by our attention within the given area.

This structure has been followed by artists and photographers for centuries to plan and proportion their works in the most harmonious and aesthetic manner possible.

“Christ in the Home of His Parents” - John Everett Millais

Moreover, many of the everyday objects that surround us are designed based on the Golden ratio proportions. Here’s a few examples:

How many more can you find?

Unlike other irrational numbers, part of the magic of the Golden ratio is that it can be defined in terms of itself. This means, it contains itself infinitely - both infinitely big and infinitely small.

This, in turn, reminds us of one of the properties of yet another fantastic mathematical construction: fractals.

Mathematics are said to be the language of the Universe, and the Golden ratio is a perfect example of how accurate this claim can be.

Whether invisible or right on-your-face, the harmonious proportions of the Golden ratio are both aesthetic and practical, a fascinating combination of art and science that will continue to astonish us and help us for many generations to come. 

Related Articles
 

Fractals


If you enjoyed this article, consider Sharing it, or Subscribe to receive similar articles once a month in your inbox.

Sources: Wikipedia, MathIsFun.com, “The Golden Section: Nature’s Greatest Secret” by Scott Olsen (Bloomsbury).